数组 和 链表是最基本的数据结构,栈、队列、树、图等复杂数据结构都是基于数组或链表方式存储
队列(Queue)特征:
-
循环队列的顺序存储是基于数组来实现的
-
队列是一种操作受限的线性表。队列只能在表的一端进行插入操作,在另一端进行删除操作。其中,允许插入的一端叫队尾,允许删除的一端叫队头。【遵守: 先进先出(First In First Out,FIFO) 规则,例如元素A、B、C、D依次入队,则出队顺序也必须是A、B、C、D】
注意咯:很多初学者都容易将 队头 和 队尾 搞混,以为元素是在队头插入在队尾删除。
循环队列特征:
-
循环队列是对队列的一种改进,主要是为了解决队尾溢出而实际上数组仍有空余空间这种“假溢出”(如图一)问题
-
循环队列的rear和front到达队尾端点,能折回数组开始处。相当于将数组首尾相连,
想象成环状(如图二)。
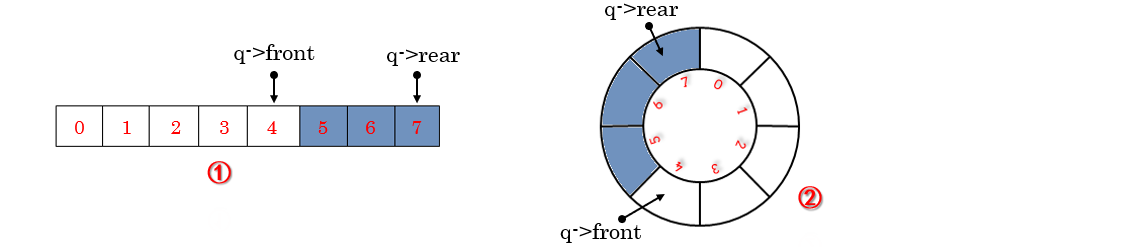
头指针Front 和 尾指针Rear:
- 循环队列中,一般头指针front指向队头元素,尾指针rear指向队尾元素的下一个元素;或者 头指针front指向队头元素的下一个元素,尾指针rear指向队尾元素。 这样的目的是满足front == rear判空条件
循环队列 - 结构体
1
2
3
4
5
6
7
8
9
10
11
12
13#define MAX_SIZE 255 //循环队列的最大容量 typedef struct C_Queue { int data[MAX_SIZE]; //指定循环队列大小 int front; //循环队列头指针 int rear; //循环队列尾指针 } *Queue; 注意:结构体后面的*Queue是指向C_Queue的结构体指针, 如果不加*,使用结构体指针需要Queue *q这样, 加了*,使用结构体指针,只需Queue q即可;
循环队列 - 创建
- 【通过malloc()函数动态创建一个循环队列】
1
2
3
4
5
6
7Queue queue_create() { Queue q = (Queue)malloc(sizeof(C_Queue)); //给循环队列分配内存空间 q->front = q->rear = 0; //初始状态下,头指针和尾指针 均指向0(下标) return q; }
循环队列 - 判空
1
2
3
4
5
6
7
8boolean queue_empty(Queue q) { if (q->front == q->rear) { //当front和rear相等时,队列为空 return TRUE; } else { return FALSE; } }
循环队列 - 判满
-
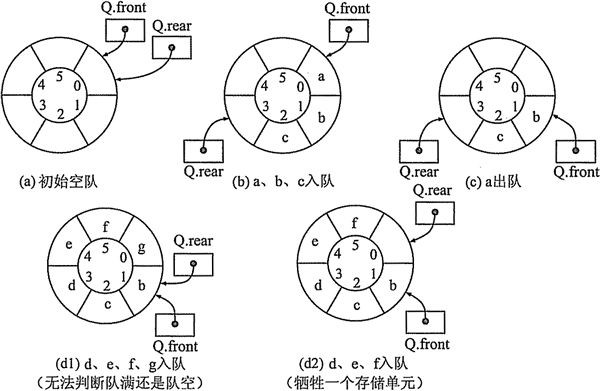
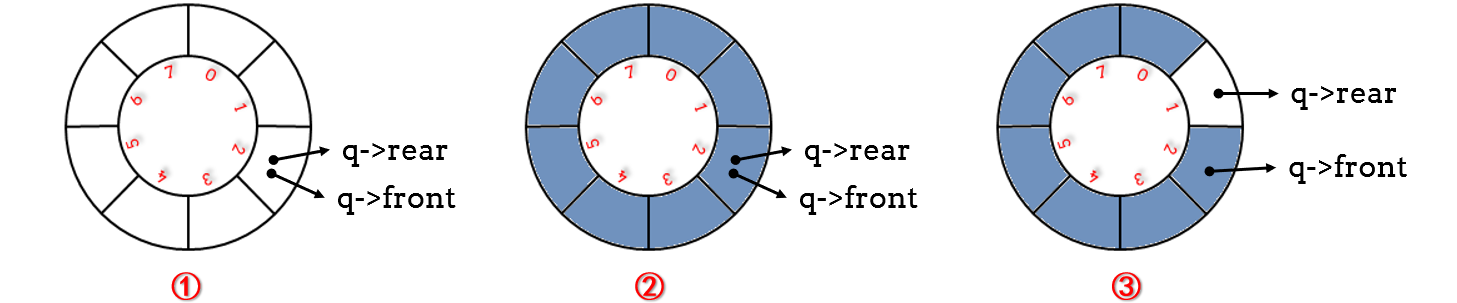
rear 和 front的值相等时(图一和图二),队列可能出现队空或队满两种情况,从而无法判别队满还是队空。
-
针对这一问题,解决方法有两种:
1. 定义一个变量size来记录当前队列的元素个数【队尾插入一个元素,size+1; 队头删除一个元素,size-1】
2. 牺牲一个存储单元(如图三),在队尾指针rear+1就能赶上队头指针front时,我们就认为队满了。【即:循环队列最大实际长度 = MAX_SIZE - 1】,这种方法的队满条件是:(q->rear+1)%MAX_SIZE == q->front 【记得%MAX_SIZE取模 ,否则可能会超出数组最大下标】,下面用的就是这种方法。
1
2
3
4
5
6
7
8boolean queue_full(Queue q) { if ((q->rear + 1) % MAX_SIZE == q->front) { return TRUE; } else { return FALSE; } }
循环队列 - 求长度
-
【由图可知:MAX_SIZE = 8,q->rear = 1,q->front = 3,队列长度length = 6】
-
验证一下:(q->rear - q->front + MAX_SIZE) % MAX_SIZE; 是不是可以求队列长度。(length = (1 - 3 + 8) % 8 = 6,没毛病)

1
2
3
4int queue_length(Queue q) { return (q->rear - q->front + MAX_SIZE) % MAX_SIZE; }
循环队列 - 入队
-
队尾插入元素,入队前要先判断循环队列是否已满
-
先将待入队的元素,插入尾指针原本指向的位置
-
尾指针rear + 1,并%MAX_SIZE取模。【模MAX_SIZE后,当尾指针指向数组下标最大值时,可以让尾指针折回数组开始处】
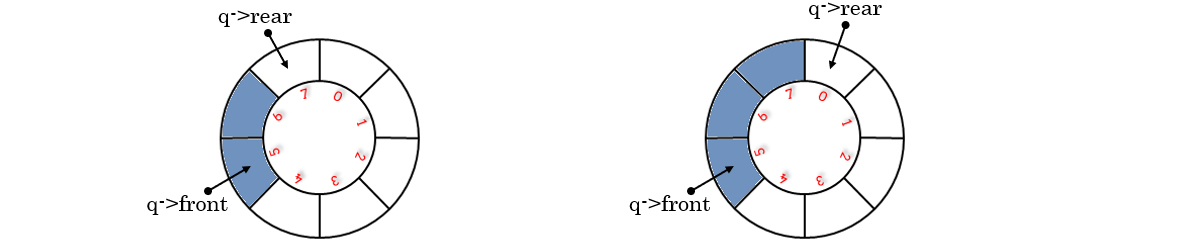
1
2
3
4
5
6
7
8
9
10
11boolean queue_rear_insert(Queue q, int x) { if (queue_full(q)) { //判断队满 printf("队列已满!n"); return FALSE; } else { q->data[q->rear] = x; //先将元素插入尾指针原本指向的位置 q->rear = (q->rear + 1) % MAX_SIZE; //尾指针+1 return TRUE; } }
循环队列 - 出队
-
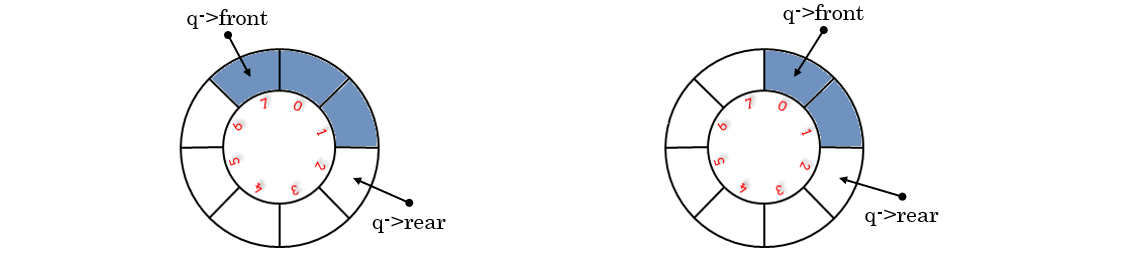
队头删除元素,出对前要先判断循环队列是否为空
-
用x记录,要出队元素的值,作为函数的返回值
-
头指针front + 1,并%MAX_SIZE取模。【模MAX_SIZE后,当头指针指向数组下标最大值时,可以让头指针折回数组开始处】
1
2
3
4
5
6
7
8
9
10
11
12int queue_front_delete(Queue q) { int x; if (queue_empty(q)) { printf("队列无元素可删除!n"); return 0; } else { x = q->data[q->front]; //用x记录头指针指向的元素值 q->front = (q->front + 1) % MAX_SIZE; //头指针front + 1,并%MAX_SIZE取模 return x; } }
循环队列 - 获取队头元素
1
2
3
4
5
6
7int queue_get_front(Queue q) { if (!queue_empty(q)) { return q->data[q->front]; } return 0; }
循环队列 - 迭代
- 将循环队列中所有元素出队,并输出
1
2
3
4
5
6
7void queue_items_printf(Queue q) { while (!(q->front == q->rear)) { printf("%d ", q->data[q->front]); //输出头指针front指向元素的值 q->front = (q->front + 1) % MAX_SIZE; //头指针front + 1 } }
整个程序代码
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
84
85
86
87
88
89
90
91
92
93
94
95
96
97
98
99
100
101
102
103
104
105
106
107
108
109
110
111
112
113
114
115
116
117
118
119
120
121
122#include <stdio.h> #include <stdlib.h> typedef enum {FALSE, TRUE} boolean; #define MAX_SIZE 8 //循环队列的最大长度 //结构体 typedef struct C_Queue { int data[MAX_SIZE]; //指定循环队列大小 int front; //循环队列头指针 int rear; //循环队列尾指针 } *Queue; //创建队列 Queue queue_create() { Queue q = (Queue)malloc(sizeof(C_Queue)); //给循环队列分配内存空间 q->front = q->rear = 0; //初始状态下,头指针和尾指针 均指向0(下标) if (q == NULL) { //内存分配失败 return NULL; } return q; } //队列判空 boolean queue_empty(Queue q) { if (q->front == q->rear) { //当front和rear相等时,队列为空 return TRUE; } else { return FALSE; } } //队列判满 boolean queue_full(Queue q) { if ((q->rear + 1) % MAX_SIZE == q->front) { return TRUE; } else { return FALSE; } } //队列长度 int queue_length(Queue q) { return (q->rear - q->front + MAX_SIZE) % MAX_SIZE; } //队尾插入元素 boolean queue_rear_insert(Queue q, int x) { if (queue_full(q)) { printf("队列已满!n"); return FALSE; } else { q->data[q->rear] = x; q->rear = (q->rear + 1) % MAX_SIZE; return TRUE; } } //队头删除元素 int queue_front_delete(Queue q) { int x; if (queue_empty(q)) { printf("队列无元素可删除!n"); return 0; } else { x = q->data[q->front]; q->front = (q->front + 1) % MAX_SIZE; return x; } } //获取队头元素 int queue_get_front(Queue q) { if (!queue_empty(q)) { return q->data[q->front]; } return 0; } //将队列所有元素出队 void queue_items_printf(Queue q) { while (!(q->front == q->rear)) { printf("%d ", q->data[q->front]); q->front = (q->front + 1) % MAX_SIZE; } } int main() { /* 创建一个循环队列 */ Queue q = queue_create(); int A[8], i; if (NULL == q) { printf("队列创建失败!n"); return -1; } printf("当前队列长度为:%dn", queue_length(q)); printf("输入要入队的元素: "); for (i = 0; i < 8; i++) { scanf("%d", &A[i]); } //将数组中的元素按顺序插入循环队列 for (i = 0; i < 8 ; i++) { queue_rear_insert(q, A[i]); } printf("当前队头元素为:%dn", queue_get_front(q)); printf("n当前队列长度为:%dn", queue_length(q)); printf("n队列元素出队顺序:"); //将循环队列所有元素出队,并输出 queue_items_printf(q); printf("n当前队列长度为:%dn", queue_length(q)); return 0; }
循环队列总结:
1. 循环队列的两个状态
-
队空状态:q->front = q->rear
-
队满状态:(q->rear + 1) % MAX_SIZE = q->front
2. 循环队列的三个操作
-
求队列长度:length = (q->rear - q->front + MAX_SIZE) % MAX_SIZE
-
元素 x 入队:q->data[q->rear] = x ; q->rear = (q->rear + 1) % MAX_SIZE ;
-
元素 x 出队:x = q->data[q->front] ; q->front = (q->front + 1) % MAX_SIZE ;
来几道题目巩固下
1. 循环队列存储在数组A[0…n]中,则入队时的操作为:__ rear = (rear+1) mod (n+1) __
- 解析:因为数组下标是从0开始的,所以数组的最大容量为n+1,即循环队列的MAX_SIZE = n+1,入队操作为:(q->rear + 1) % MAX_SIZE = (rear + 1) mod (n + 1) 【mod是%的意思,N mod M = N % M】
2. 已知循环队列的存储空间为数组A[21],front指向队头元素的前一个位置,rear指向队尾元素,假设当前front和rear的值分别为8和3,则该队列的长度为:__ 16 __
- 解析:length = (q->rear - q->front + MAX_SIZE) % MAX_SIZE = (3 - 8 + 21) % 21 = 16
循环队列JAVA语言实现
最后
以上就是悦耳大地最近收集整理的关于循环队列 (顺序存储)的全部内容,更多相关循环队列内容请搜索靠谱客的其他文章。








发表评论 取消回复