Poisson Equation
Contents[hide]
|
Poisson's Equation[edit]
Definition[edit]
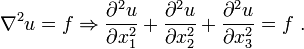
Description[edit]
Appears in almost every field of physics.
Solution to Case with 4 Homogeneous Boundary Conditions[edit]
Let's consider the following example, where 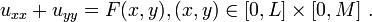 and the Dirichlet boundary conditions are as follows:
and the Dirichlet boundary conditions are as follows:

In order to solve this equation, let's consider that the solution to the homogeneous equation will allow us to obtain a system of basis functions that satisfy the given boundary conditions. We start with the Laplace equation: 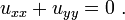
Step 1: Separate Variables[edit]
Consider the solution to the Poisson equation as 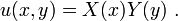 Separating variables as in the solution to the Laplace equation yields:
Separating variables as in the solution to the Laplace equation yields:


Step 2: Translate Boundary Conditions[edit]
As in the solution to the Laplace equation, translation of the boundary conditions yields:
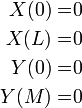
Step 3: Solve Both SLPs[edit]
Because all of the boundary conditions are homogeneous, we can solve both SLPs separately.
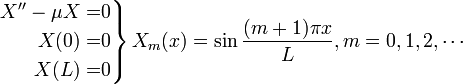
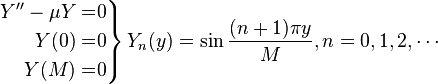
Step 4: Solve Non-homogeneous Equation[edit]
Consider the solution to the non-homogeneous equation as follows:
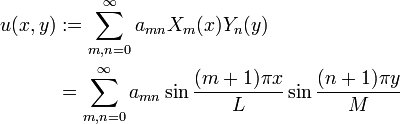
We substitute this into the Poisson equation and solve:
![begin{align}f(x,y)& = u_{xx}+u_{yy} \& = sum_{m,n=0}^infty left { a_{mn}left lbrack -frac{(m+1)^2pi^2}{l^2} right rbrack sin frac{(m+1)pi x}{l} sin frac{(n+1)pi y}{m} right } +left { a_{mn}left lbrack -frac{(n+1)^2pi^2}{m^2} right rbrack sin frac{(m+1)pi x}{l} sin frac{(n+1)pi y}{m} right } \& = sum_{m,n=0}^infty underbrace{left [ -a_{mn} left ( frac{(m+1)^2pi^2}{l^2}+frac{(n+1)^2pi^2}{m^2} right ) right ]}_{a_{mn}} sin frac{(m+1)pi x}{l} sin frac{(n+1)pi y}{m}end{align}](http://upload.wikimedia.org/wikiversity/en/math/0/7/4/07408f441e6fc6895088fc6abecdce83.png)
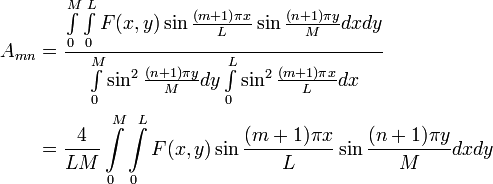
![a_{mn}=-frac{4}{lmleft [ frac{(m+1)^2pi^2}{l^2} + frac{(n+1)^2pi^2}{m^2} right ]}intlimits_0^m int limits_0^l f(x,y) sin frac{(m+1)pi x}{l} sin frac{(n+1)pi y}{m} dx dy; m,n=0,1,2,cdots](http://upload.wikimedia.org/wikiversity/en/math/a/8/6/a86e5fa0962705d94653ccbb92985b33.png)
Solution to General Case with 4 Non-homogeneous Boundary Conditions[edit]
Let's consider the following example, where 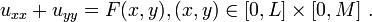 and the boundary conditions are as follows:
and the boundary conditions are as follows:

The boundary conditions can be Dirichlet, Neumann or Robin type.
Step 1: Decompose Problem[edit]
For the Poisson equation, we must decompose the problem into 2 sub-problems and use superposition to combine the separate solutions into one complete solution.
- The first sub-problem is the homogeneous Laplace equation with the non-homogeneous boundary conditions. The individual conditions must retain their type (Dirichlet, Neumann or Robin type) in the sub-problem:
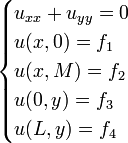
- The second sub-problem is the non-homogeneous Poisson equation with all homogeneous boundary conditions. The individual conditions must retain their type (Dirichlet, Neumann or Robin type) in the sub-problem:
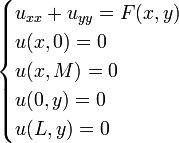
Step 2: Solve Subproblems[edit]
Depending on how many boundary conditions are non-homogeneous, the Laplace equation problem will have to be subdivided into as many sub-problems. The Poisson sub-problem can be solved just as described above.
Step 3: Combine Solutions[edit]
The complete solution to the Poisson equation is the sum of the solution from the Laplace sub-problem 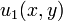 and the homogeneous Poisson sub-problem
and the homogeneous Poisson sub-problem 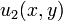 :
:

来源: http://en.wikiversity.org/wiki/Poisson_Equation
最后
以上就是无辜发夹最近收集整理的关于Poisson Equation方程求解--转载Poisson Equation 的全部内容,更多相关Poisson内容请搜索靠谱客的其他文章。








发表评论 取消回复