原文档： SHA-3 Standard: Permutation-Based Hash and Extendable-Output Functions，《SHA-3标准：基于置换的哈希与可扩展输出函数》

1 INTRODUCTION

2012年10月，NIST选用Keccak作为SHA-3的标准算法，经过对原算法的修改，于2015年8月发布标准文档FIPS 202，作为对FIPS 180-4 SHA-2的补充。

准确地说，Keccak团队当初提交的是Keccak-f[1600]。

SHA-3 family：

• 4 cryptographic hash functions：
  • SHA3-224
  • SHA3-256
  • SHA3-384
  • SHA3-512
• 2 eXtendable-Output Functions (XOFs)：
  • SHAKE128 (Secure Hash Algorithm KECCAK)
  • SHAKE256

其中，XOF输出的哈希值可以任意指定长度。

消息后面会追加2 bits，用来区分hash函数和XOFs，或者以后的sha3变体。

2 GLOSSARY

3 KECCAK-p Permutations

2 parameters:

• b, in {25, 50, 100, 200, 400, 800, 1600}: the fixed length of the strings that are permuted, called the width of the permutation.
• n_r, any positive integer: the number of iterations of an internal transformation, called a round.

表示：KECCAK-p[b, n_r]

b取值25和50仅仅用于研究原理，不可实际应用。

3.1 State

对于KECCAK-p[b, n_r] permutation, state表示b bits.

另外两个参数：

• w = b/25
• l = log₂(b/25) = log₂w

b	25	50	100	200	400	800	1600
w	1	2	4	8	16	32	64
l	0	1	2	3	4	5	6

比特流state（S）也可以表示为 5x5xW的三维bit数组A[x][y][z](0≤x<5, 0≤y<5, and 0≤z<w)，这里还涉及以下概念：

• 二维：sheets, planes, and slices
• 一维：rows, columns, and lanes.

3.1.2 Converting Strings to State Arrays

A[x,y,z] = S[w(5y+x)+z]

其实就是点线面体，顺序为：

1. lane ，z线
2. plane ，xz面
3. state，垒高

eg. b=1600, w=64

A[4,2,63]=S[959]

3.1.3 Converting State Arrays to Strings

其实就是反过来的过程，以lane为单位，从左到右，从下往上。

还是以b=1600, w=64为例：

S = A[0, 0, 0] || A[0, 0, 1] || ... || A[0, 0, 63]
 || A[1, 0, 0] || ... || A[1, 0, 63]
 || A[4, 4, 0] || ... || A[4, 4, 63]

3.1.4 Labeling Convention for the State Array

Z坐标为0的第一个slice面的中心为state原点。

X和Y轴坐标为3,4,0,1,2，也就是说S[0]对应(3,3,0)。

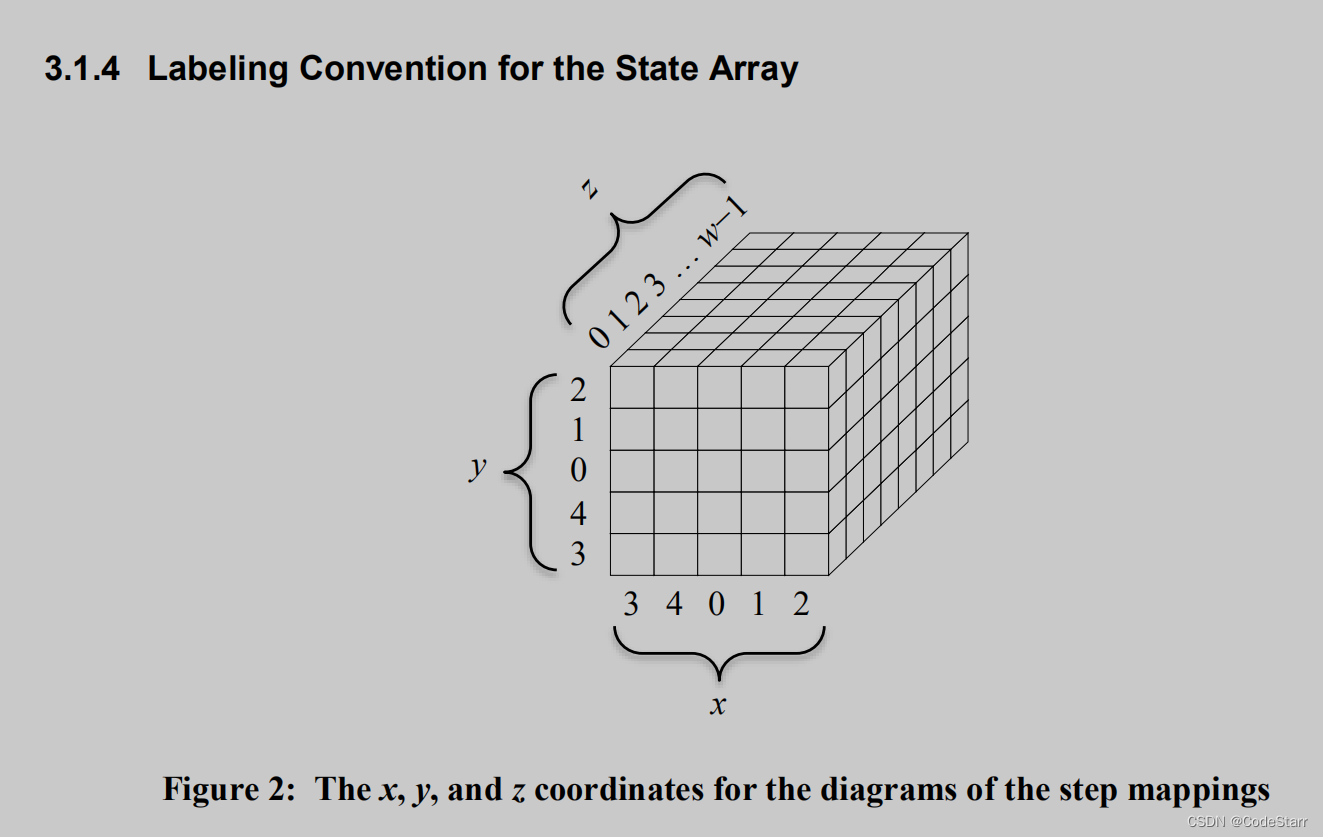
虽然中间的lane坐标为（0，0），但结合3.1.2和3.1.3，从代码的角度看，可以通过对5取余来处理，也就是说把数组第一个元素当成中间的lane即可。

3.2 Step Mappings

step mapping == Rnd == A round of a KECCAK-p permutation == round function，由5个函数组成。

构成Rnd（A round of a KECCAK-p permutation）的5个函数:

 θ, ρ, π, χ, ι
 or
 theta, rho, pi, chi, iota

• 输入参数：state array A
• 返回：state array A’

iota额外需要一个round index参数 i_r。

这一部分会比较绕，而且是以bit为单位进行讲解，但在代码中则是以ullLane（8字节unsigned long long）为单位进行运算。

theta

C[x,z]=A[x,0,z] 
	^ A[x,1,z] 
	^ A[x,2,z] 
	^ A[x,3,z] 
	^ A[x,4,z]
D[x,z]=C[(x-1) mod 5, z] 
	^ C[(x+1) mod 5, (z-1) mod w]
A′[x,y,z] = A[x, y, z] ^ D[x,z]

C：将5个plane面进行异或，在代码层面会得到5个ullLane（1个plane）；

D：将column两两异或，在代码层面，相当于将8字节整型向高地址移位（小端整型，所以左移），得到的仍是5个ullLane；

将每个plane与plane D中异或后返回state array。

rho

这个函数的作用是将(0,0,z)以外的lane进行循环位移，offset表如下：

	x = 3	x = 4	x = 0	x = 1	x = 2
y = 2	153	231	3	10	171
y = 1	55	276	36	300	6
y = 0	28	91	0	1	190
y = 4	120	78	210	66	253
y = 3	21	136	105	45	15

因为(0,0,z)这个中间的lane不需要变化，所以一共移动24个lane：

x,y = (1,0)
d = {}
for t in range(24):	# 23 times
    d[t] = (x,y)
    x,y = (y, (2*x + 3*y)%5)    # 这个公式恰好遍历24个lane
print(len(d))   # 24

for t,v in d.items():
    print(v)
    # z = (z - (t+1)*(t+2) /2) % w
    # 以下省略w
    print("t=%d offset = %dn" % (t,(-(t+1)*(t+2)/2)))
# 24
# (1, 0)
# t=0 offset = -1
# (0, 2)
# t=1 offset = -3
# (2, 1)
# t=2 offset = -6
# (1, 2)
# t=3 offset = -10
# (2, 3)
# t=4 offset = -15
# (3, 3)
# t=5 offset = -21
# (3, 0)
# t=6 offset = -28
# (0, 1)
# t=7 offset = -36
# (1, 3)
# t=8 offset = -45
# (3, 1)
# t=9 offset = -55
# (1, 4)
# t=10 offset = -66
# (4, 4)
# t=11 offset = -78
# (4, 0)
# t=12 offset = -91
# (0, 3)
# t=13 offset = -105
# (3, 4)
# t=14 offset = -120
# (4, 3)
# t=15 offset = -136
# (3, 2)
# t=16 offset = -153
# (2, 2)
# t=17 offset = -171
# (2, 0)
# t=18 offset = -190
# (0, 4)
# t=19 offset = -210
# (4, 2)
# t=20 offset = -231
# (2, 4)
# t=21 offset = -253
# (4, 1)
# t=22 offset = -276
# (1, 1)
# t=23 offset = -300

计算出来的偏移为负值，对于unsigned long long小端存储类型来说是左移，与表格中的正值都是向z轴的正方向移动。

pi

该函数的作用是将中心以外的各个lane进行重新排列（乾坤大挪移 嘿哈）。

# A'[x,y] = A[(x + 3*y) % 5, x]
x,y = (1,0)
s = set()
for t in range(24):
    s.add((x,y))
    print(x,y)
    x,y = (x + 3*y) % 5, x	# 这个公式也恰好遍历24个lane
print(len(s))	# 24

chi

该函数是对每个row进行置换：

A′[x,y,z] = A[x,y,z] 
	^ ( (~A[(x+1) % 5, y, z])
		& A[(x+2) % 5, y, z])

当然在代码层面，还是以lane为单位进行操作的。

而且对于pi和chi两个置换函数，不要直接对原state进行操作，需要拷贝副本。

iota

这一函数的目的是使(0,0)这个lane与 round constant进行异或，因为这个lane受round index影响，而另外24个lane不会经过iota函数处理。

如前所说，iota函数需要一个round index参数，即3.3部分的Keccak_p函数的循环索引。

伪代码如下：

keccak_state iota(keccak_state A, uint32_t ir)
{
	// ir = Algorithm7(b, nr)  round index
	keccak_state retA = A;
	// uint32_t w = b / 25;	// 64
	// uint32_t l = log2(w);	// 6
	// uint64_t RC[w] = {0};	//  round constant
	// uint32_t j = 0;
	for (j = 0; j <= l; ++j)
	{
		RC[2 * *j - 1] = rc(j + 7 * ir);
        // the last one is RC[w-1]
	}
    // for all 0 <= z <= w
	retA[0, 0, z] = retA[0, 0, z] ^ RC[z];
	return retA;
}

可以看出，RC数组其实是固定的，并不需要在运行时计算，硬编码即可：

#define NUMBER_OF_ROUNDS 24
static const uint64_t roundconstants[NUMBER_OF_ROUNDS] = {
    0x0000000000000001ULL,    0x0000000000008082ULL, 
    0x800000000000808aULL,    0x8000000080008000ULL, 
    0x000000000000808bULL,    0x0000000080000001ULL, 
    0x8000000080008081ULL,    0x8000000000008009ULL, 
    0x000000000000008aULL,    0x0000000000000088ULL, 
    0x0000000080008009ULL,    0x000000008000000aULL, 
    0x000000008000808bULL,    0x800000000000008bULL, 
    0x8000000000008089ULL,    0x8000000000008003ULL, 
    0x8000000000008002ULL,    0x8000000000000080ULL, 
    0x000000000000800aULL,    0x800000008000000aULL, 
    0x8000000080008081ULL,    0x8000000000008080ULL, 
    0x0000000080000001ULL,    0x8000000080008008ULL
};

对于SHA3中每个lane，长度为8字节（64 bits），其中可置为1的下标为代码中的2 * *j - 1，如下：

0x80000000 8000808B

至于生成它的函数rc(t)，根据keccak原文档 1.2节，它其实是一个线性反馈移位寄存器（LFSR）：

// rc[t] = (x^t mod x^8 + x^6 + x^5 + x^4 + 1) mod x in GF(2)[x].

int LFSR86540(uint8_t* LFSR)
{
	int result = ((*LFSR) & 0x01) != 0;
	if (((*LFSR) & 0x80) != 0)
		// Primitive polynomial over GF(2): x^8+x^6+x^5+x^4+1
		(*LFSR) = ((*LFSR) << 1) ^ 0x71;	// 0111 0001
	else
		(*LFSR) <<= 1;
	return result;
}

void KeccakInitializeRoundConstants()
{
	uint8_t LFSRstate = 0x01;
	unsigned int ir, j, bitPosition;
	uint8_t nr = 24;
	uint64_t KeccakRoundConstants[64] = {0};
	for (ir = 0; ir < nr; ir++) {
		KeccakRoundConstants[ir] = 0;
		for (j = 0; j < 7; j++) {
			bitPosition = (1 << j) - 1; //2^j-1
			if (LFSR86540(&LFSRstate))
				KeccakRoundConstants[ir] ^= (uint64_t)1 << bitPosition;
		}
	}
	for (ir = 0; ir < 24; ++ir)
	{
		printf("%016llxn", KeccakRoundConstants[ir]);
	}
}

3.3 KECCAK-p[b, n_r]

Rnd函数：

Rnd(A, ir) = iota(chi(pi(rho( theta(A) ))), ir)

Keccak-p[b, n_r]有n_r轮Rnd迭代，如下：

string Keccak_p(string s/*length b*/,int nr)	// Algorithm 7
{
	string s;
    keccak_state A = convertS2A(s);
    uint32_t w = b / 25;	// 64
	uint32_t l = log2(w);	// 6
    for(int ir = 12+2*l-nr; ir <= 12+2*l-1; ++ir)
    {
        A = Rnd(A, ir);
    }
    s = convertA2S(A);
    return s;
}

在代码中，s是单字节数组，state array则是ull类型数组，所以convertS2A也是按照小端存储来转换。

沿着z轴方向，相当于从低地址到高地址。

3.4 KECCAK-f

原版本keccak中定义了KECCAK-f ，它其实是n_r==12+2l时的keccak-p，也是SHA3最终选用的操作:

KECCAK-f[b] = KECCAK-p[b, 12+2*l]

SHA3选用了比特流长度b==1600，即KECCAK-p[1600， 24]，相当于是KECCAK-f[1600]

4 SPONGE CONSTRUCTION

Keccak采用的算法结构叫做海绵结构。

string Z = SPONGE[f, pad, r](string N, uint d)

3 components:

• f :
  • An underlying function on fixed-length strings;
  • b is called the width of f;
  • In SHA-3, f is invertible, although the sponge construction does not require f to be invertible.
• r:
  • A parameter called the rate
  • capacity，c == b - r，specified in Sec. 5.2
• pad: A padding rule
  • m = len(N), (m + len(pad(r, m)) ) mod r == 0

2 inputs:

• N: a bit string
• d: bit length of the output string

Output:

• string Z of length d

海绵结构的流程（对应代码中的final函数）可看作以下几步：

1. pad
2. absorbing 吸入，string–>State Array，
3. 轮函数执行f()，即keccak-f()，且每“吸入” 1个分组（rate 字节）就执行一次
4. squeezing 挤出，State Array–>string of length d，而且每“挤出”1个分组，就要再执行一次f()。

在代码中第2、3步相当于hash udpate。

海绵结构和rate，这俩词非常形象，笔者一开始把rate理解为valid buffer，因为这些数据其实是存储在block中的，只不过不像sha2那样把block缓冲区填满。函数f相当于挤压海绵，吸收数据和输出摘要，都需要“挤压”一下。

根据第7章，SHA-3 hash分组大小rate如下表：

Hash	SHA3-224	SHA3-256	SHA3-384	SHA3-512
BlockSize(bytes)	144	136	104	72

5.1 Specification of pad10*1

The padding rule for KECCAK is called multi-rate padding.

inputs:

• r : rate
• m : len(N)

output:

• string P, (m + len(pad(r, m)) ) mod r == 0，即填充完后，数据长度是r的倍数，所以r就是分组大小。

uint pad10x1(uint r, uint m)
{
    uint P;
    uint j = (-m-2) % r;
            
	// (m + len(P)) % r == 0
    // len(P) == (-m) % r
    P = (1<<j) + 1;	// 1 || 0*j || 1
    return P;
}

实际的补充方法，请参见附录B。

5.2 Specification of KECCAK[c]

根据第4节海绵结构，总结一下keccak的组成：

• 海绵函数Keccak-p[b, 12+2l]
• 填充规则pad10*1
• rate（<b），其实就是分组大小

如3.1所述，比特流长度b有7个可选值。当选用最大值b==1600时，就只有rate不确定了。而rate就由c（capacity）决定了：

KECCAK[c](N, d) = SPONGE[
	f = KECCAK-p[1600, 24]
	,pad = pad10*1
	,r = 1600–c](N, d)

6 SHA-3 FUNCTION SPECIFICATIONS

6.1 SHA-3 Hash Functions

SHA3有4个基于KECCAK[c] 定义的hash函数：

SHA3-224(M) = KECCAK[448] (M || 01, 224)
SHA3-256(M) = KECCAK[512](M || 01, 256)
SHA3-384(M) = KECCAK[768] (M || 01, 384)
SHA3-512(M) = KECCAK[1024](M || 01, 512)

如第1部分所述，消息后面会追加2 bits，用来区分hash函数和XOFs。

所以SHA3的这4个hash算法，相当于给消息追加01后的Keccak-f[1600]。

另外可以观察到，c==2d，也就是说，capacity是输出摘要长度的两倍。

6.2 SHA-3 Extendable-Output Functions

SHA3的两个XOF，SHAKE128 和 SHAKE256，也是基于KECCAK[c] 定义的：

SHAKE128(M, d) = KECCAK[256] (M || 1111, d)
SHAKE256(M, d) = KECCAK[512] (M || 1111, d)

6.3 Alternate Definitions of SHA-3 Extendable-Output Functions

还有两个原版sponge functions, RawSHAKE128 和 RawSHAKE256：

RawSHAKE128(J, d) = KECCAK[256] (J || 11, d)
RawSHAKE256(J, d) = KECCAK[512] (J || 11, d)

这个11后缀用以区分RawSHAKE和SHA3 SHAKE

当J = M || 11时，就等价于SHAKE128和SHAKE256。这个11后缀，则是用来兼容RawSHAKE和Sakura coding scheme。

A Security

A.1 Summary

Function	Output Size	Collision	Preimage	2nd Preimage
SHA3-224	224	112	224	224
SHA3-256	256	128	256	256
SHA3-384	384	192	384	384
SHA3-512	512	256	512	512
SHAKE128	d	min(d/2, 128)	≥ min(d, 128)	min(d, 128)
SHAKE256	d	min(d/2, 256)	≥ min(d, 256)	min(d, 256)

其中，Collision，Preimage，2nd Preimage为抵抗攻击的安全级别。

B Examples

B.1 Conversion Functions

这里给出了二进制和十六进制的转换法。简单地说，在每个字节中bit是小端存储。

以0xA32E为例：

# support trunc
def h2b(h:int, n:int):
    ret = ""
    b = bin(h)[2:]
    print(b)	# 10100011 00101110
    nBy = len(b) // 8
    for i in range(nBy):
        ret += b[8*i:8*i+8][-1::-1]
        ret += " "
    return ret[:n];
# 0xA32E为大端存储 "xA3x2E"
print(h2b(0xA32E, 14))
# 11000101 01110

B.2 Hexadecimal Form of Padding Bits

以上运算是以bit为单位的，但在实际应用中，数据N以及rate肯定是以字节为单位，也就是说r和m均为8的倍数。

在原文档附录B中，给出了以下公式，q为需要填补的字节数：

// m单位: byte
q = (r/8) - (m % (r/8))

这个很好理解，就是补充字节满足整体长度为rate的倍数，等价于下面这个公式：

# 单位: byte
8q == r - 8m % r

5.1 填充方法部分是以bit为单位讲解的，而在代码中，则是以字节为单位，更准确地说是十六进制。

结合5.1和6.1，要添加的bit流如下：

01 1 0j 1

也就是说，要填充的0的个数j，可能是4+8*n。填充结束后，还要将每个字节的bit顺序反转，如下：

def pad(j:int):
    strRet = "0x"
    padding = "01"
    padding += "1" + "0" * j + "1"
    nBy = len(padding) // 8
    for i in range(nBy):
        tmp = padding[8*i: 8*i+8][::-1]
        strRet += "%02x" % (int(tmp, 2))
    return strRet
print(pad(4))
# 0x86	q == 1
print(pad(12))
# 0x0680 q == 2
print(pad(20))
# 0x060080 q == 3
print(pad(28))
# 0x06000080 q == 4

而对于XOF，则是这样填充：

# 1111 1 0j 1
def pad_xof(j:int):
    strRet = "0x"
    padding =  "1111"
    padding += "1" + "0" * j + "1"
    nBy = len(padding) // 8
    for i in range(nBy):
        tmp = padding[8*i: 8*i+8][::-1]
        strRet += "%02x" % (int(tmp, 2))
    return strRet
print(pad(2))
# 0x9f	q == 1
print(pad(10))
# 0x1f80	q == 2
print(pad(18))
# 0x1f0080	q == 3
print(pad(26))
# 0x1f000080	q == 4

实现

https://github.com/C0deStarr/CryptoImp/tree/main/Hash/sha/:

• sha3.h
• sha3.c

参考资料

• 原文档 https://nvlpubs.nist.gov/nistpubs/FIPS/NIST.FIPS.202.pdf

• https://csrc.nist.gov/publications/detail/fips/202/final

• Keccak Team

• 原版Keccak：http://keccak.noekeon.org/Keccak-reference-3.0.pdf

• http://keccak.noekeon.org/Sakura.pdf

• 海绵结构：http://sponge.noekeon.org/CSF-0.1.pdf

最后

以上就是彪壮烤鸡为你收集整理的SHA3算法笔记1 INTRODUCTION2 GLOSSARY3 KECCAK-p Permutations4 SPONGE CONSTRUCTION6 SHA-3 FUNCTION SPECIFICATIONSA SecurityB Examples实现参考资料的全部内容，希望文章能够帮你解决SHA3算法笔记1 INTRODUCTION2 GLOSSARY3 KECCAK-p Permutations4 SPONGE CONSTRUCTION6 SHA-3 FUNCTION SPECIFICATIONSA SecurityB Examples实现参考资料所遇到的程序开发问题。

如果觉得靠谱客网站的内容还不错，欢迎将靠谱客网站推荐给程序员好友。