LeetCode 2087. 网格图中机器人回家的最小代价（脑筋急转弯）

81 阅读 0 评论 54 点赞

我是靠谱客的博主火星上未来，最近开发中收集的这篇文章主要介绍LeetCode 2087. 网格图中机器人回家的最小代价（脑筋急转弯），觉得挺不错的，现在分享给大家，希望可以做个参考。

概述

文章目录

- 1. 题目
- 2. 解题

1. 题目

给你一个 m x n 的网格图，其中 (0, 0) 是最左上角的格子，(m - 1, n - 1) 是最右下角的格子。
给你一个整数数组 startPos ，startPos = [startrow, startcol] 表示初始有一个机器人在格子 (startrow, startcol) 处。
同时给你一个整数数组 homePos ，homePos = [homerow, homecol] 表示机器人的家在格子 (homerow, homecol) 处。

机器人需要回家。
每一步它可以往四个方向移动：上，下，左，右，同时机器人不能移出边界。
每一步移动都有一定代价。
再给你两个下标从 0 开始的额整数数组：长度为 m 的数组 rowCosts 和长度为 n 的数组 colCosts 。

如果机器人往上或者往下移动到第 r 行的格子，那么代价为 rowCosts[r] 。
如果机器人往左或者往右移动到第 c 列的格子，那么代价为 colCosts[c] 。

请你返回机器人回家需要的 最小总代价 。

示例 1：
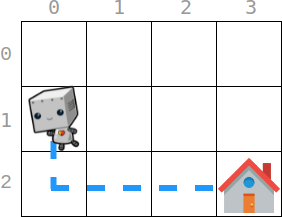
在这里插入图片描述

输入：startPos = [1, 0], homePos = [2, 3], rowCosts = [5, 4, 3], colCosts = [8, 2, 6, 7]
输出：18
解释：一个最优路径为：
从 (1, 0) 开始
-> 往下走到 (2, 0) 。代价为 rowCosts[2] = 3 。
-> 往右走到 (2, 1) 。代价为 colCosts[1] = 2 。
-> 往右走到 (2, 2) 。代价为 colCosts[2] = 6 。
-> 往右走到 (2, 3) 。代价为 colCosts[3] = 7 。
总代价为 3 + 2 + 6 + 7 = 18

示例 2：
输入：startPos = [0, 0], homePos = [0, 0], rowCosts = [5], colCosts = [26]
输出：0
解释：机器人已经在家了，所以不需要移动。总代价为 0 。
 
提示：
m == rowCosts.length
n == colCosts.length
1 <= m, n <= 10^5
0 <= rowCosts[r], colCosts[c] <= 10^4
startPos.length == 2
homePos.length == 2
0 <= startrow, homerow < m
0 <= startcol, homecol < n

来源：力扣（LeetCode）
链接：https://leetcode-cn.com/problems/minimum-cost-homecoming-of-a-robot-in-a-grid
著作权归领扣网络所有。商业转载请联系官方授权，非商业转载请注明出处。

2. 解题

首先不管怎么走不能走到，起点和终点构成的矩形之外，会增加额外的花费
然后在上面条件下，不论怎么走，按照两个方向的分量来看，花费都是一样的：行的花费+列的花费

class Solution {
public:
    int minCost(vector<int>& startPos, vector<int>& homePos, vector<int>& rowCosts, vector<int>& colCosts) {
        int ans = 0;
        if(homePos[0] >= startPos[0])
            for(int i = startPos[0]+1; i <= homePos[0]; ++i)
                ans += rowCosts[i];
        else
            for(int i = startPos[0]-1; i >= homePos[0]; --i)
                ans += rowCosts[i];
        if(homePos[1] >= startPos[1])
            for(int i = startPos[1]+1; i <= homePos[1]; ++i)
                ans += colCosts[i];
        else
            for(int i = startPos[1]-1; i >= homePos[1]; --i)
                ans += colCosts[i];
        return ans;
    }
};