一、参考资料
买卖股票的最佳时机II
https://programmercarl.com/0122.%E4%B9%B0%E5%8D%96%E8%82%A1%E7%A5%A8%E7%9A%84%E6%9C%80%E4%BD%B3%E6%97%B6%E6%9C%BAII.html
跳跃游戏
https://programmercarl.com/0055.%E8%B7%B3%E8%B7%83%E6%B8%B8%E6%88%8F.html
跳跃游戏 II
https://programmercarl.com/0045.%E8%B7%B3%E8%B7%83%E6%B8%B8%E6%88%8FII.html
二、LeetCode122.买卖股票的最佳时机II
https://leetcode.cn/problems/best-time-to-buy-and-sell-stock-ii/description/
给你一个整数数组 prices ,其中 prices[i] 表示某支股票第 i 天的价格。
在每一天,你可以决定是否购买和/或出售股票。你在任何时候 最多 只能持有 一股 股票。你也可以先购买,然后在 同一天 出售。
返回 你能获得的 最大 利润 。
示例 1:
输入:prices = [7,1,5,3,6,4] 输出:7 解释:在第 2 天(股票价格 = 1)的时候买入,在第 3 天(股票价格 = 5)的时候卖出, 这笔交易所能获得利润 = 5 - 1 = 4 。 随后,在第 4 天(股票价格 = 3)的时候买入,在第 5 天(股票价格 = 6)的时候卖出, 这笔交易所能获得利润 = 6 - 3 = 3 。 总利润为 4 + 3 = 7 。
示例 2:
输入:prices = [1,2,3,4,5] 输出:4 解释:在第 1 天(股票价格 = 1)的时候买入,在第 5 天 (股票价格 = 5)的时候卖出, 这笔交易所能获得利润 = 5 - 1 = 4 。 总利润为 4 。
示例 3:
输入:prices = [7,6,4,3,1] 输出:0 解释:在这种情况下, 交易无法获得正利润,所以不参与交易可以获得最大利润,最大利润为 0 。
把利润分解为每天为单位的维度,而不是从0天到第3天整体去考虑!
那么根据prices可以得到每天的利润序列:(prices[i] - prices[i - 1]).....(prices[1] - prices[0])
class Solution:
def maxProfit(self, prices: List[int]) -> int:
res = 0
for i in range(1, len(prices)):
res += max(prices[i] - prices[i - 1], 0)
return res动态规划:
class Solution:
def maxProfit(self, prices: List[int]) -> int:
length = len(prices)
dp = [[0] * 2 for _ in range(length)]
dp[0][0] = -prices[0]
dp[0][1] = 0
for i in range(1, length):
dp[i][0] = max(dp[i-1][0], dp[i-1][1] - prices[i]) #注意这里是和121. 买卖股票的最佳时机唯一不同的地方
dp[i][1] = max(dp[i-1][1], dp[i-1][0] + prices[i])
return dp[-1][1]三、LeetCode55. 跳跃游戏
https://leetcode.cn/problems/jump-game/description/
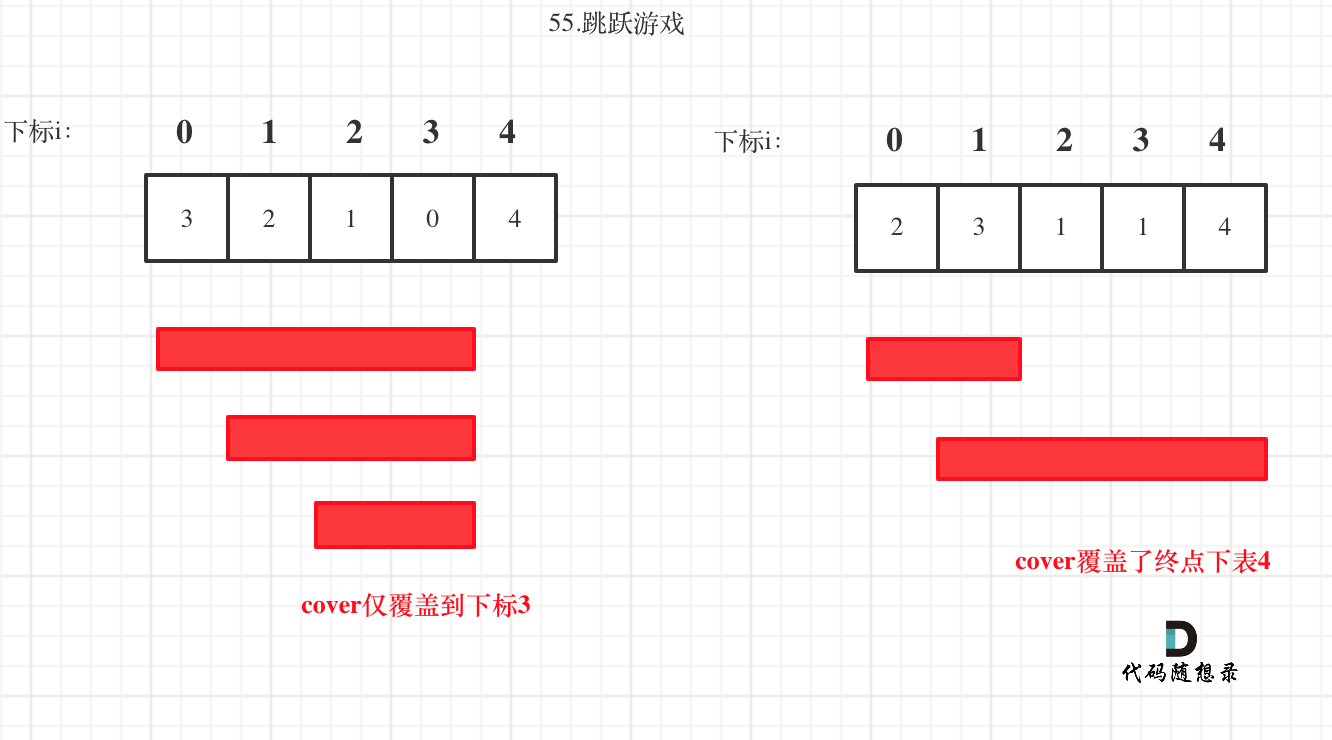
给定一个非负整数数组 nums ,你最初位于数组的 第一个下标 。
数组中的每个元素代表你在该位置可以跳跃的最大长度。
判断你是否能够到达最后一个下标。
示例 1:
输入:nums = [2,3,1,1,4] 输出:true 解释:可以先跳 1 步,从下标 0 到达下标 1, 然后再从下标 1 跳 3 步到达最后一个下标。
示例 2:
输入:nums = [3,2,1,0,4] 输出:false 解释:无论怎样,总会到达下标为 3 的位置。但该下标的最大跳跃长度是 0 , 所以永远不可能到达最后一个下标。
提示:
1 <= nums.length <= 3 * 104
0 <= nums[i] <= 105
思路:
这个问题就转化为跳跃覆盖范围究竟可不可以覆盖到终点!
每次移动取最大跳跃步数(得到最大的覆盖范围),每移动一个单位,就更新最大覆盖范围。
贪心算法局部最优解:每次取最大跳跃步数(取最大覆盖范围),整体最优解:最后得到整体最大覆盖范围,看是否能到终点。
class Solution:
def canJump(self, nums: List[int]) -> bool:
cover = 0
if (len(nums) == 1): return True
for i in range(len(nums)):
# 每次只能在cover范围内移动
if i <= cover:
cover = max(i + nums[i], cover)
if cover >= len(nums) - 1:
return True
return False四、LeetCode45.跳跃游戏II
https://leetcode.cn/problems/jump-game-ii/
给定一个长度为 n 的 0 索引整数数组 nums。初始位置为 nums[0]。
每个元素 nums[i] 表示从索引 i 向前跳转的最大长度。换句话说,如果你在 nums[i] 处,你可以跳转到任意 nums[i + j] 处:
0 <= j <= nums[i]
i + j < n
返回到达 nums[n - 1] 的最小跳跃次数。生成的测试用例可以到达 nums[n - 1]。
示例 1:
示例 2:
输入: nums = [2,3,0,1,4] 输出: 2
提示:
1 <= nums.length <= 104
0 <= nums[i] <= 1000
题目保证可以到达 nums[n-1]
版本一:
class Solution {
public:
int jump(vector<int>& nums) {
if (nums.size() == 1) return 0;
int curDistance = 0; // 当前覆盖最远距离下标
int ans = 0; // 记录走的最大步数
int nextDistance = 0; // 下一步覆盖最远距离下标
for (int i = 0; i < nums.size(); i++) {
nextDistance = max(nums[i] + i, nextDistance); // 更新下一步覆盖最远距离下标
if (i == curDistance) { // 遇到当前覆盖最远距离下标
if (curDistance < nums.size() - 1) { // 如果当前覆盖最远距离下标不是终点
ans++; // 需要走下一步
curDistance = nextDistance; // 更新当前覆盖最远距离下标(相当于加油了)
if (nextDistance >= nums.size() - 1) break; // 下一步的覆盖范围已经可以达到终点,结束循环
} else break; // 当前覆盖最远距到达集合终点,不用做ans++操作了,直接结束
}
}
return ans;
}
};Python版本:
class Solution:
def jump(self, nums: List[int]) -> int:
if len(nums) == 1: return 0
ans = 0
curDistance = 0
nextDistance = 0
for i in range(len(nums)):
nextDistance = max(i + nums[i], nextDistance)
if i == curDistance:
if curDistance != len(nums) - 1:
ans += 1
curDistance = nextDistance
if nextDistance >= len(nums) - 1: break
return ans
# 贪心版本二
class Solution:
def jump(self, nums: List[int]) -> int:
if len(nums) == 1:
return 0
curDistance, nextDistance = 0, 0
step = 0
for i in range(len(nums)-1):
nextDistance = max(nextDistance, nums[i]+i)
if i == curDistance:
curDistance = nextDistance
step += 1
return step今日总结:
不焦虑也不自卑,不过是有一腔热血和勇敢的心
继续加油哈小赵~
最后
以上就是甜甜指甲油最近收集整理的关于代码随想录算法训练营第三十二天 | 122.买卖股票的最佳时机II,55. 跳跃游戏,45.跳跃游戏II的全部内容,更多相关代码随想录算法训练营第三十二天内容请搜索靠谱客的其他文章。








发表评论 取消回复