Codeforces #369（Div.2）B.Chris and Magic Square【思维】

62 阅读 0 评论 41 点赞

我是靠谱客的博主帅气荷花，最近开发中收集的这篇文章主要介绍Codeforces #369（Div.2）B.Chris and Magic Square【思维】，觉得挺不错的，现在分享给大家，希望可以做个参考。

概述

ZS the Coder and Chris the Baboon arrived at the entrance of Udayland. There is a n × n magic grid on the entrance which is filled with integers. Chris noticed that exactly one of the cells in the grid is empty, and to enter Udayland, they need to fill a positive integer into the empty cell.

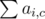Chris tried filling in random numbers but it didn't work. ZS the Coder realizes that they need to fill in a positive integer such that the numbers in the grid form a magic square. This means that he has to fill in a positive integer so that the sum of the numbers in each row of the grid ( $\text{[math]}$ ), each column of the grid ( $\text{[math]}$ ), and the two long diagonals of the grid (the main diagonal — 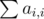$\text{[math]}$ and the secondary diagonal — 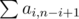$\text{[math]}$ ) are equal.

Chris doesn't know what number to fill in. Can you help Chris find the correct positive integer to fill in or determine that it is impossible?

Input

The first line of the input contains a single integer n (1 ≤ n ≤ 500) — the number of rows and columns of the magic grid.

n lines follow, each of them contains n integers. The j-th number in the i-th of them denotes ai, j (1 ≤ ai, j ≤ 109 or ai, j = 0), the number in the i-th row and j-th column of the magic grid. If the corresponding cell is empty, ai, j will be equal to 0. Otherwise, ai, j is positive.

It is guaranteed that there is exactly one pair of integers i, j (1 ≤ i, j ≤ n) such that ai, j = 0.

Output

Output a single integer, the positive integer x (1 ≤ x ≤ 1018) that should be filled in the empty cell so that the whole grid becomes a magic square. If such positive integer x does not exist, output - 1 instead.

If there are multiple solutions, you may print any of them.

Note

In the first sample case, we can fill in 9 into the empty cell to make the resulting grid a magic square. Indeed,

The sum of numbers in each row is:

4 + 9 + 2 = 3 + 5 + 7 = 8 + 1 + 6 = 15.

The sum of numbers in each column is:

4 + 3 + 8 = 9 + 5 + 1 = 2 + 7 + 6 = 15.

The sum of numbers in the two diagonals is:

4 + 5 + 6 = 2 + 5 + 8 = 15.

In the third sample case, it is impossible to fill a number in the empty square such that the resulting grid is a magic square.

题目大意：

给你一个n*n的矩阵，其中有一个空位（0来表示），让你找一个方案，在空位上填上一个（1-1e18）的数，使得每行每列和主对角线上的和相同，如果找不到方案，输出-1.

思路：

1、首先判断每一行的和是否相等，再判断每一列的和是否相等（当然要排除0所在的行和列），如果每一行和每一列的和都相等了，然后我们直接累加0所在的行的值，然后模拟赋给这个位子上值：sum（sum表示每行的和）-累加和。

2、然后这个时候我们就能够保证了每一行每一列的和是全部相等的了，然后对于两条主对角线判断一下是否相等即可，如果相等，并且赋值的数是（1-1e18）就输出出来，否则输出-1.

3、注意输出的解需要是1-1e18区间内的解才行，另外，注意特判一下n==1的时候的输出，再另外，注意一下数据类型。

Ac代码：

#include<stdio.h>
#include<string.h>
using namespace std;
#define ll __int64
ll a[505][505];
int main()
{
    int n;
    while(~scanf("%d",&n))
    {
        int row;
        int col;
        for(int i=1; i<=n; i++)
        {
            for(int j=1; j<=n; j++)
            {
                scanf("%I64d",&a[i][j]);
                if(a[i][j]==0)
                {
                    row=i;
                    col=j;
                }
            }
        }
        if(n==1)
        {
            printf("1n");
            continue;
        }
        int flag=0;
        ll rowsum=-1;
        for(int i=1; i<=n; i++)
        {
            if(i==row)continue;
            ll tmp=0;
            for(int j=1; j<=n; j++)
            {
                tmp+=a[i][j];
            }
            if(rowsum==-1)rowsum=tmp;
            else
            {
                if(rowsum!=tmp)flag=1;
            }
        }
        if(flag==1)
        {
            printf("-1n");
            continue;
        }
        for(int i=1; i<=n; i++)
        {
            if(i==col)continue;
            ll tmp=0;
            for(int j=1; j<=n; j++)
            {
                tmp+=a[j][i];
            }
            if(tmp!=rowsum)flag=1;
        }
        if(flag==1)
        {
            printf("-1n");
            continue;
        }
        /*----------*/
        ll tmp=0;
        for(int i=1; i<=n; i++)
        {
            tmp+=a[row][i];
        }
        if(rowsum-tmp>=1)
        a[row][col]=rowsum-tmp;
        else
        {
            printf("-1n");
            continue;
        }
        ll tmpp=0;
        for(int i=1;i<=n;i++)
        {
            tmpp+=a[i][i];
        }
        if(tmpp!=rowsum)
        {
            printf("-1n");
            continue;
        }
        tmpp=0;
        for(int i=n; i>=1; i--)
        {
            tmpp+=a[i][n-i+1];
        }
        if(tmpp!=rowsum)
        {
            printf("-1n");
            continue;
        }
        printf("%I64dn",a[row][col]);
    }
}